|
|
|
|
||||
Raise one hand as high over your head as you can. Keep it up there as you read this article!
Raising your hand will let you physically experience some of the ideas
that physicists hold about energy, work, and power, the subjects I'll
be talking about in this article. Virtually everything you do -- whether
physical or mental, by hand or by machine, active or sedentary --
involves energy. To a physicist, life is really a complex series of
energy transactions, in which energy is transformed from one form
to another or transferred from one object to another.
Take,
for example, an apple tree. The tree absorbs light energy from sunlight,
converting the light energy into chemical potential energy stored
in chemical bonds. The tree uses this energy to build leaves and
branches and fruit. When an apple falls from the tree to the ground,
its energy of position (stored as gravitational potential energy)
is converted to kinetic energy, the energy of motion, as it falls.
When the apple hits the ground, kinetic energy is transformed into
heat energy. When you eat the apple, your body converts its chemical
energy into the movement of your muscles. If your muscles are tired,
and you decide to drive your car instead of walking, the engine
of your car takes gasoline (which contains chemical energy stored
long ago by plants) and converts it into heat. Then it takes that
heat and converts it into motion, or kinetic energy.
So far I've used the word energy a bundle of times. What is energy? Unfortunately, it isn't really that easy to define. So we'll have to talk a bit more before we can get a handle on it.
Hey,
how's your arm?
Getting tired yet? Your arm, of course, weighs something. When you
hold it over your head, you have to exert a force to support it, and
you get tired. You might even describe this activity as "a lot of
work." A physicist, however, would disagree. It isn't that physicists
are callous (some are and some aren't). But when physicists talk about
work, they are talking about something very specific that they call
mechanical work. A physicist defines work (W) as the force (F) multiplied
by the distance (d) over which it is exerted. This is expressed as
W = F x d. Work is measured in units called joules (pronounced "jools").
When
you hold your arms over your head, you aren't moving anything. But
mechanical work involves both force and distance, and there isn't
any distance. Ergo, you're doing no mechanical work. Zero. Nada.
Of course you're certainly doing what might be called "biological work." If you stand for a period of time with your arms upraised, you will eventually have to eat another peanut butter sandwich to replenish the energy you are consuming through the metabolic process. But physicists don't talk about biological work; they talk about mechanical work. And they talk about energy, which they define as "the ability to do work." So there you have it -- your definition of energy! It may seem a little devious or circular, and not very satisfying. Defining this stuff we call energy is difficult, because energy isn't something you can get your hands on. It's an idea, a concept, an abstraction. To quote a college textbook: "Energy is not a 'thing' or a 'substance,' but a concept that has been developed to describe in specific terms how fast something is moving, where it is, how hot it is, and so on. . . . Energy is really an abstraction, a mental bookkeeping device that we have invented." (Robert Romer, Energy: An Introduction to Physics. Freeman, 1976, pp. 3, 89.) I realize that this may muddy, rather than clarify, the definition of energy for you at this point. But don't throw up your hands in despair Oops! . . . I forgot! . . . you've already got one of them up there! OK, you can put your arm down.
That's the good news. But don't relax! There's bad news, too. Since
holding your arm up doesn't qualify as work to a physicist, you're
not off the hook yet. In fact, you're about to do some real mechanical
work. That is, you will exert a force on an object which will move
the object a certain distance. And in the process, you'll learn the
difference between work, energy, and power.
Got a
couple of two-liter soda bottles around? If they're empty, just
fill them with water --it won't make any difference whether they
hold water or soda for what you're going to do with them. Screw
on the caps and put the bottles on the floor. Now pick them up,
one in each hand, and lift them up one meter (about three feet)
in one second (the time it takes you to say "one-thousand-one").
Do it again. And again.
The bottles always have the same weight, and you always lift them the same distance. That means that each time you lift them you always do the same amount of "work." (Remember our definition of work: force multiplied by distance, or W = F x d.) It doesn't matter whether you lift them rapidly or slowly; it's the same amount of work. But if you lift them rapidly, you are doing the work in a shorter period of time. That's what physicists call power. Energy is the ability to do work, but power is the rate at which you do it. In physics-ese, power (P) is equal to work (W) divided by time (t), or P = W/t. Therefore, when you lift the bottles faster, your work is the same, but your power is greater. Here's another way to understand the difference between energy and power. Suppose you had $30. You could choose to spend it all in one day, or you could spend $1 each day for thirty days. Either way, you end up spending the same amount of money. But in the first case your rate of spending was high ($30/day), while in the second case your rate of spending was low ($1/day). The total amount of money ($30) is analogous to energy or work, and the rate of spending ($30/day or $1/day) is analogous to power. Energy and work are both measured in joules. Power is measured in joules per second, a unit that we call a watt. When you repeatedly lifted the bottles one meter in one second, you experienced what it felt like to expend energy at the same rate as a 40 watt light bulb.2 Since the power of an average laborer's daily work has been calculated at 75 watts, you should be able to raise and lower the bottles virtually all day long.
What does all this have to do with the kind of energy that your local
utility company delivers to your home? Well, envision a huge building
with row upon row of benches filled with people just like yourself.
In fact, imagine 25,000 such individuals. But instead of lifting soda
bottles, they're turning cranks by exerting about the same amount
of effort. (Since they're still exerting a force through a distance,
they're still doing work.) If these cranks were connected to electrical
generators, then you would be looking at a 1 megawatt (1 million watts)
powerhouse.
Ever
been inside a powerhouse? How do you know that this isn't the way
your electricity is generated? That's how the Romans made their
ships move: by people power. The power expended by each galley slave
could be rated in watts, as could the power of a modern-day member
of a crew in a racing shell, or any person rowing a boat. Or walking.
Or riding a bicycle. Even as you read this, you radiate heat at
about 100 watts.
But wait a minute. When you get your monthly utility bill, you don't get charged for joules or watts. Your bill tells you how many kilowatt-hours of electricity you used, and how many therms of gas. What gives? One kilowatt-hour means you are using energy at the rate of 1 kilowatt (1000 watts, or 1000 joules per second) for a period of 1 hour. An hour is 3,600 seconds. If you use 1000 joules each second for an hour, that's 3,600,000 joules. So 1 kilowatt-hour is equivalent to 3,600,000 joules. Do you see why your utility company prefers kilowatt-hours? Charging by the joule would be kind of like buying gasoline for your car by the drop rather than by the gallon. Of course, they could just as easily call a kilowatt-hour 3.6 megajoules (3.6 mJ) or 3,600 kilojoules (3,600 kJ). And now for your gas bill, which is in therms! Wait! Don't leave! A therm is a unit used by utilities to stand for approximately 100 million joules. So if you used 10 therms of gas, you've used 1 billion joules! I t's time to plug in
Your public utility generates electricity by burning a fossil
fuel (coal, oil, or natural gas), operating a nuclear reactor, or
using water, wind, or solar energy. For simplicity, we'll limit
our discussion to fossil fuels. But similar stories, with different
details, could be told for the other energy sources.
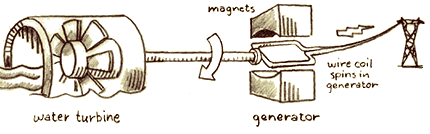
In the
fossil fuel plant, oil, coal, or natural gas is burned and the potential
energy stored in the chemical bonds of the fuel is transformed into
heat energy, which boils water and produces steam. The steam turns
the turbine of an electrical generator, and the heat energy is transformed
into the kinetic energy of the spinning turbine. The generator spins
coils of wire in a magnetic field, transforming its kinetic energy
into electrical energy. The electricity is sent through wires to
your house. Once there, it can be transformed into heat in your
toaster or kinetic energy in your fan or used to run a myriad of
other electrical devices.
This
principle is one of the cornerstones of physics. It provides a way
to link some very diverse phenomena. How is a baseball speeding
toward home plate like a candle flame? How can you compare either
of those to a gallon of gas or, for that matter, the sandwich you
had for lunch? The kinetic energy of the baseball, the thermal energy
of the flame, the chemical potential energy contained in the gasoline
and the sandwich can all be measured in joules and can all be transformed
into work. If any of these forms of energy is transformed into another
form, you'll have the same amount of energy after the transformation
as you did before. The concept of energy -- and the realization
that energy cannot be created or destroyed -- has allowed physicists
to recognize the relationships among things that seem, at first
glance, to be unrelated, a step toward understanding the essential
unity of nature.
|
|
|||||
|
copyright Exploratorium 2001 |
||||||

 Understanding
Energy & Power
Understanding
Energy & Power

 So
watt?
So
watt?
 Since we're
talking about your utility bill, did you ever wonder where the electricity
in your house comes from? The easy answer is "from the plug," of course.
But where does the plug get it? For a closing shot at clarifying the
concept of energy itself, we'll consider your electricity as an example
of energy and its transformations.
Since we're
talking about your utility bill, did you ever wonder where the electricity
in your house comes from? The easy answer is "from the plug," of course.
But where does the plug get it? For a closing shot at clarifying the
concept of energy itself, we'll consider your electricity as an example
of energy and its transformations.
 The crucial, overriding principle in this series of energy transformations
(and in all energy transactions) is that energy may change form,
but it can't be created or destroyed. In the textbook definition
I cited earlier, energy was associated with bookkeeping. What's
amazing about this bookkeeping is that it always balances. If you
add up all the energy that's around after an energy transformation,
you always end up with the same amount of energy you started with,
though the form may have changed.
The crucial, overriding principle in this series of energy transformations
(and in all energy transactions) is that energy may change form,
but it can't be created or destroyed. In the textbook definition
I cited earlier, energy was associated with bookkeeping. What's
amazing about this bookkeeping is that it always balances. If you
add up all the energy that's around after an energy transformation,
you always end up with the same amount of energy you started with,
though the form may have changed.